The notes on a sheet of music show a musician which frequencies to play and when. Why do the same notes played on different instruments sound so different?

The reason the same musical note sounds different when played on various instruments is because the harmonic overtones and envelope of each instrument is unique. When a frequency is played, other frequencies, called harmonics, are created. Each instrument has a unique harmonic character. The duration of the notes, or envelope, played on different instruments also varies widely.

In this post, you’ll learn what harmonic character and envelope are and what causes them. You’ll also see graphical representations of notes played on different instruments to help visualize how the sound from each differs.

The reason the same musical note sounds different when played on various instruments is because the harmonic overtones and envelope of each instrument is unique. 

What Is The Sound Of An Instrument?

If you already have a good grasp on what sound is on a physical level, you can feel free to move on to the next section. If you are new to the science behind sound, or just want a refresher, this section will briefly explain the two main elements behind every sound we hear.

For a more in-depth explanation of audio basics, check out this Audio University article. It goes into more detail and contains animations that are helpful for visualizing sound waves.

Frequency

Frequency is closely related to pitch. Generally speaking, high-pitched notes are high in frequency and low-pitched notes are low in frequency. If you’re new to the concept, you might be asking, “Frequency of what?”. 

Every sound we hear is a series of vibrations. Let’s take a loudspeaker for example.

When no sound is being sent through the speaker, it rests in its default position. We will call this starting position “0”. When an audio signal is sent to the speaker, it starts to push outward and pull inward. You can see this in the following image.

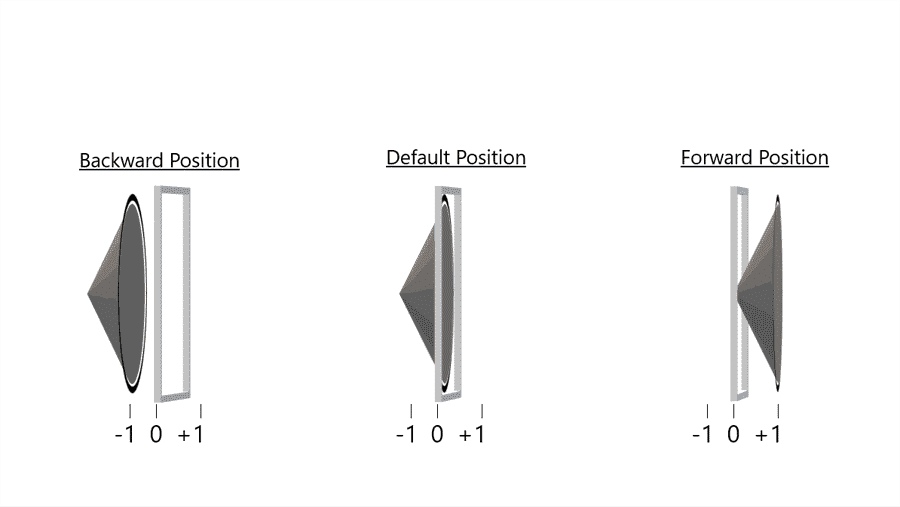
You can see a much more detailed animation of how a speaker works by Animagraffs. This is a website that creates amazing 3D models of various technologies.

As a speaker vibrates forward and backward, it displaces the air particles in the atmosphere. This creates a chain reaction through the air from the speaker to our ears. 

Each time the speaker moves from the default position, to the forward position, to the backward position and returns to the default position, one cycle is completed.

Frequency is the number of cycles completed per second. It is measured in Hertz (Hz). 

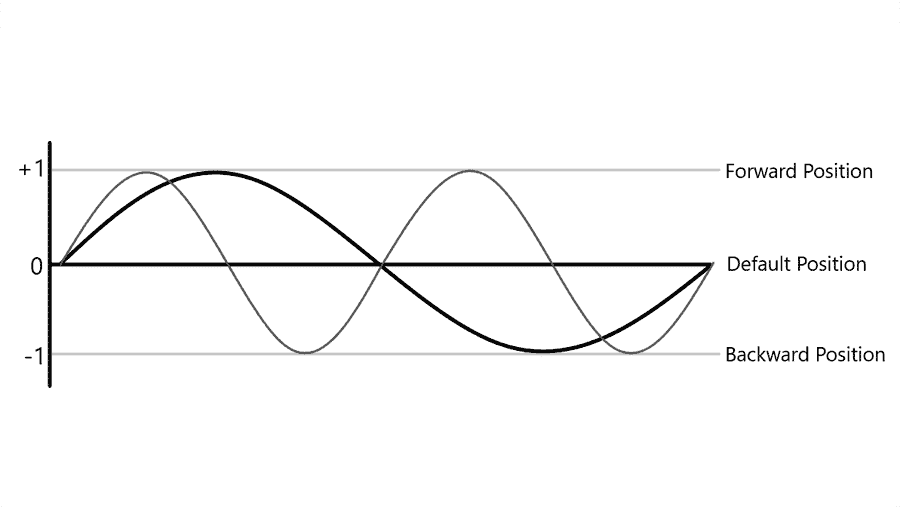
The following image shows one cycle of movement represented on a graph.

The frequency of the musical note A is 440Hz, meaning the cycle above is completed 440 times per second when this note is played. This is the A in the middle octave of a piano, called A4. The frequency of the A in the next octave up is 880Hz. This pattern continues with each octave: 440Hz, 880Hz, 1760Hz, 3520Hz, 7040Hz, and so on. 

The frequency of a note doubles with each octave. 

This graph shows a frequency along with its first octave.

Amplitude

The amplitude of a sound determines the force of the sound wave. Generally speaking, the higher the amplitude, the louder the sound. 

In the examples above, the amplitude of the wave is an arbitrary unit of 1. Amplitude is measured in pascals, a unit of pressure.

For the purpose of this post, it is only important that you understand the relationship between amplitude and loudness.

If you want to get a more in-depth understanding of these concepts, read this post about how sound works and the properties of a sound wave.

Why Do Instruments Sound Different?

The same sheet of music played by a different instrument will sound radically different, even though the same notes are played. This is because each instrument has its own unique tone, or timbre. 

In this section, we will explore the elements that make up an instrument’s timbre.

The Harmonic Character Of The Instrument

Harmonics are overtones created when a note, or frequency, is played. 

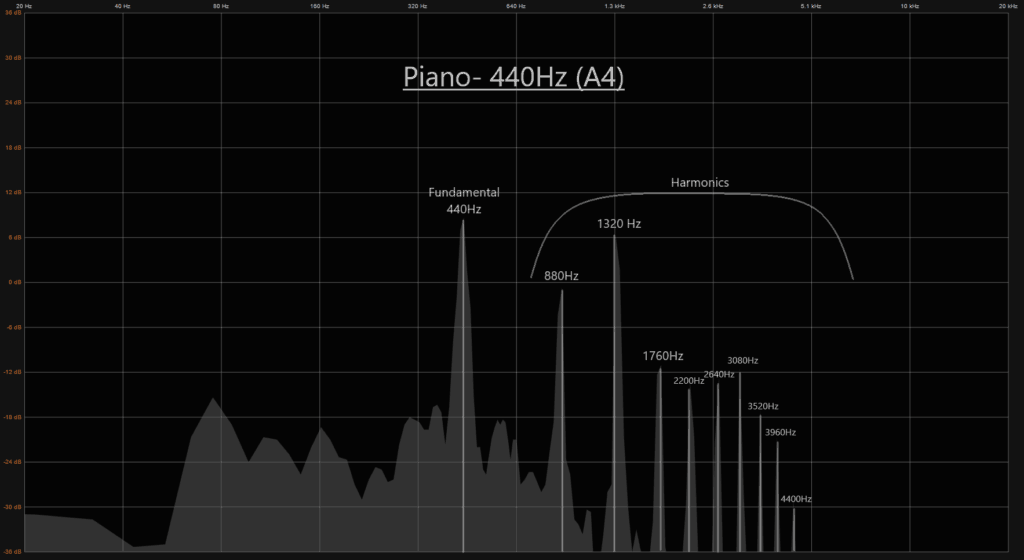
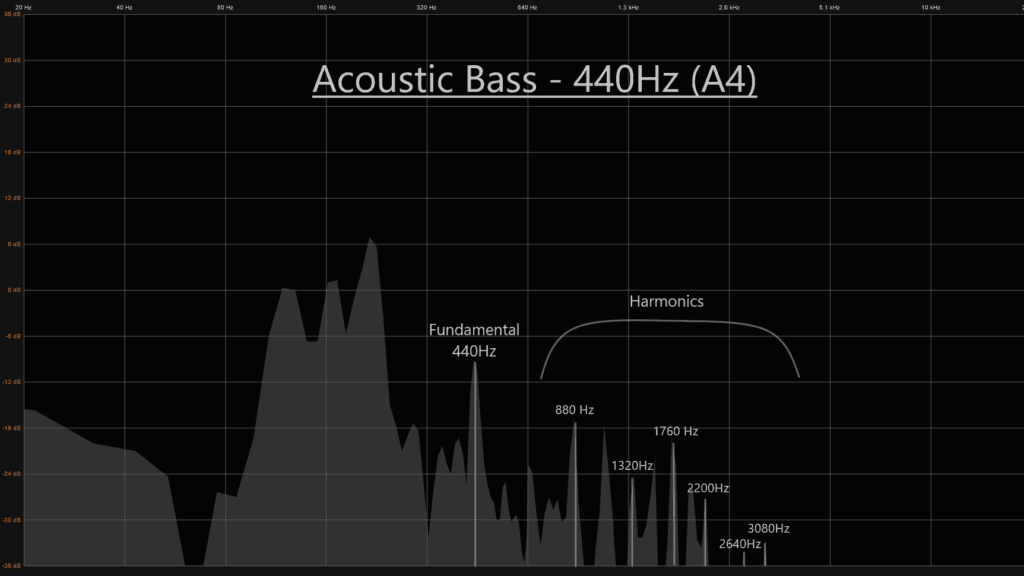
When the musical note A is played on an instrument, a sound wave is created with a frequency of 440Hz. This is the fundamental frequency, or first harmonic. 

Harmonics are calculated by adding the fundamental frequency to itself again and again. 

The second harmonic of 440Hz is 880Hz, an octave above the fundamental frequency (440Hz + 440Hz = 880Hz). The third harmonic of 440Hz is 1320Hz (440Hz + 440Hz + 440Hz = 1320Hz). The cycle continues, adding the fundamental frequency each time:

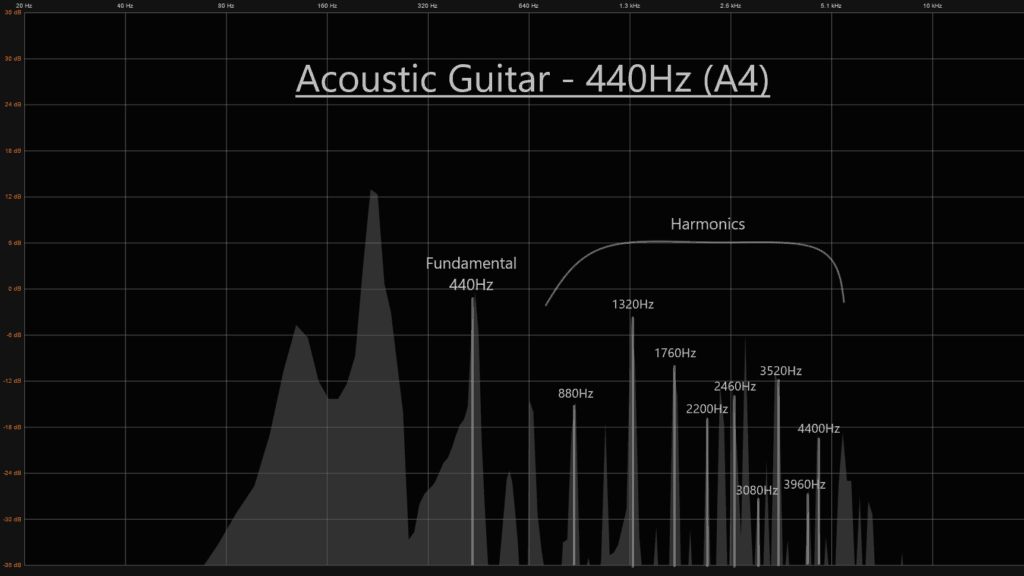
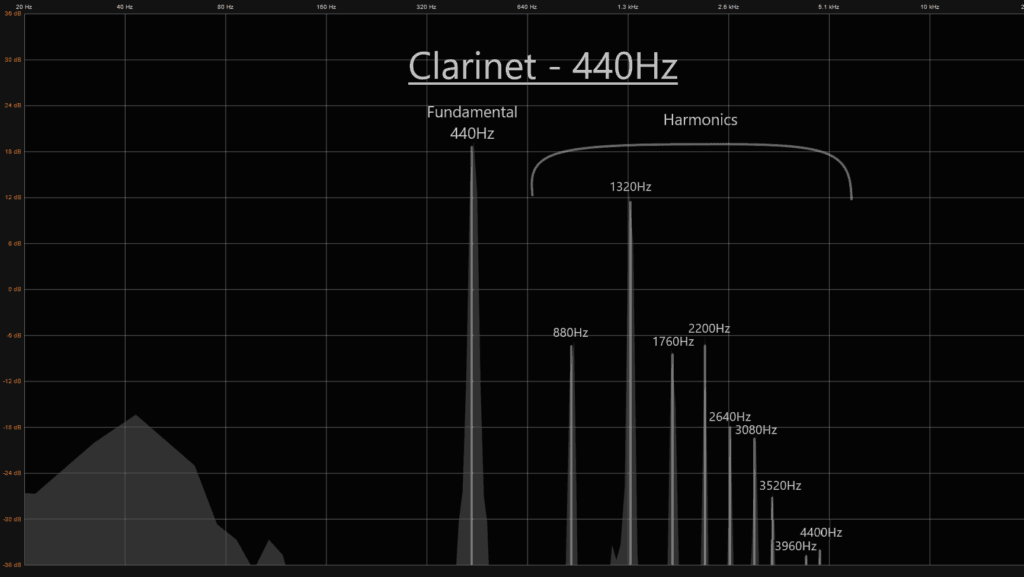
Each instrument that vibrates at 440Hz will also vibrate at these same harmonic frequencies. The difference between instruments is how loud each harmonic is in comparison to the fundamental frequency. 

The following graphs show the frequencies created when the same note is played on various instruments. Notice that the same frequencies are present, but the intensity of these frequencies varies depending on the specific instrument.

Why Do Harmonics Exist?

Fundamental and harmonic frequencies are determined by the dimensions of a sound source. 

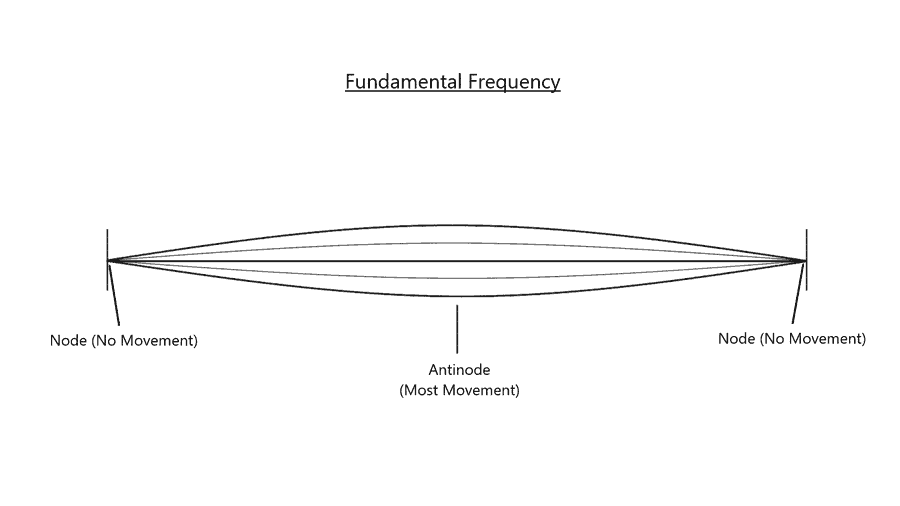
This can be understood visually using the image of a guitar string.

As the string vibrates at its fundamental frequency, it is held in place at each end. There is no motion at the nodes and the greatest motion in the antinodes. The vibration at each frequency is represented in the following images.

In reality, strings vibrate at all of the following frequencies to varying degrees, simultaneously.

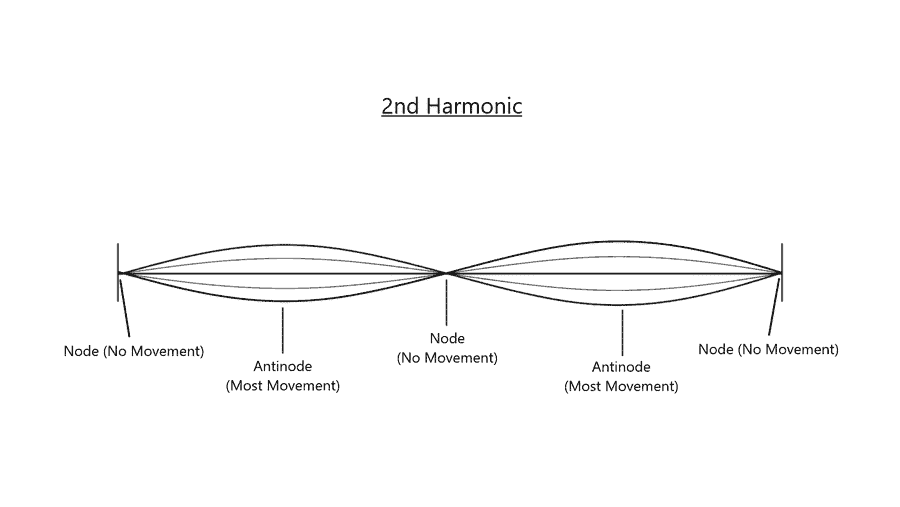
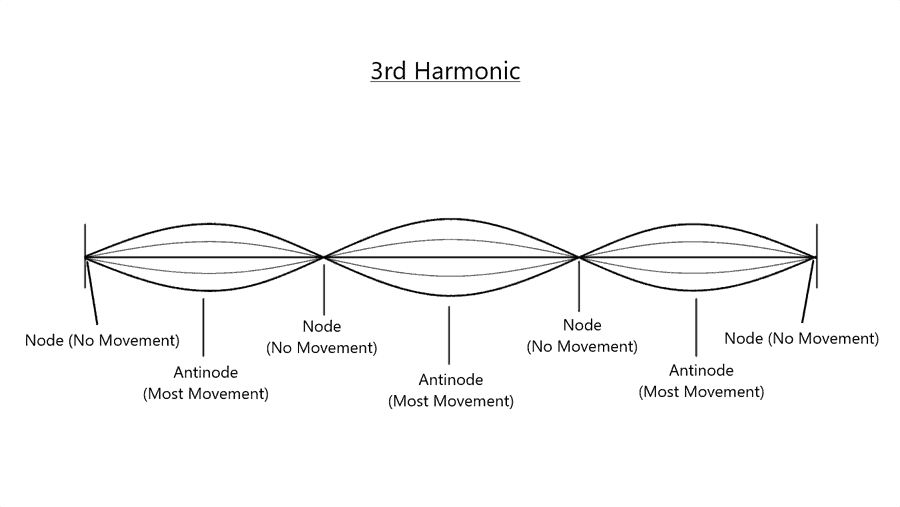
The harmonic sequence continues far beyond the 3rd harmonic. 

Each harmonic varies in amplitude. The unique mix of these harmonic frequencies determines a string’s harmonic character.

The harmonic character is what gives a guitar string its unique timbre.

The louder the harmonics, the brighter the sound. The quieter the harmonics, the duller the sound.

The sound differences between different acoustic guitars using the same strings, however, has to do with the body shape and density of the guitars. 

These factors, shape and density, determine the harmonic character of all instruments. 

Take a look at the following video. This is called a Chladni plate.

A speaker is mounted below the plate, causing the plate to vibrate at a certain fundamental frequency. Granules of sand are spread over the plate. The granules settle in the sections of the plate that do not vibrate.

The Chladni plate allows us to visualize the harmonic character of differently shaped plates! Check it out:

If you are enjoying this post, you should consider joining the email list at the bottom of this page. I’m passionate about audio and it seems that you are too! If you have friends who would find Audio University interesting, please share on social media!

OK… Let’s continue on to the other element of an instrument’s unique sound – envelope.

The Envelope Of The Instrument

In addition to a unique harmonic character, every instrument has a unique envelope.

The envelope of an instrument describes the level variation of a note or chord over time. It is divided into three parts: Attack, Sustain, and Decay. 

Although the envelope of an electronically synthesized instrument is described by attack, decay, sustain, and release, the purpose of this section is to explore the variables that determine the envelope of acoustic instruments.

Attack

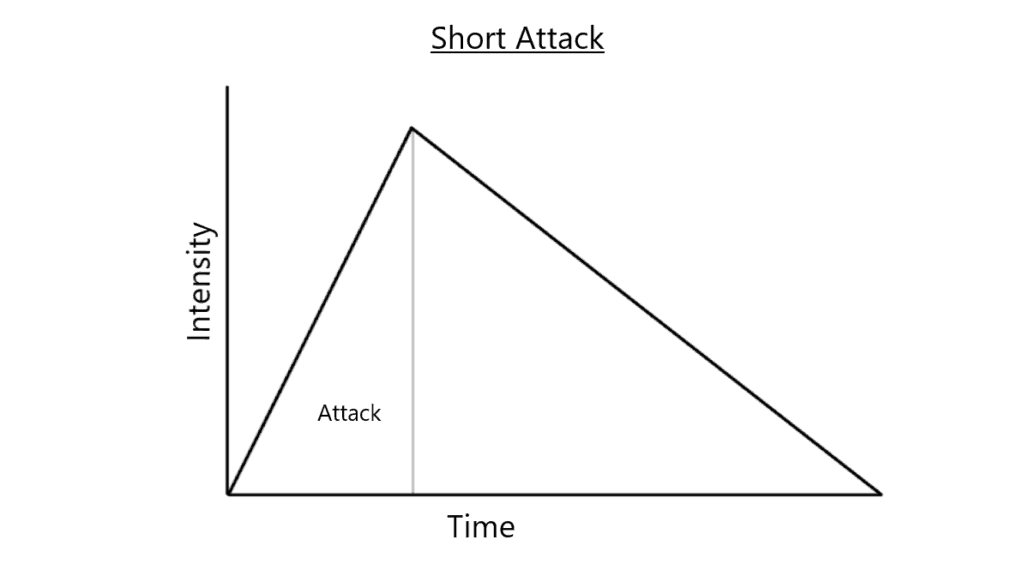
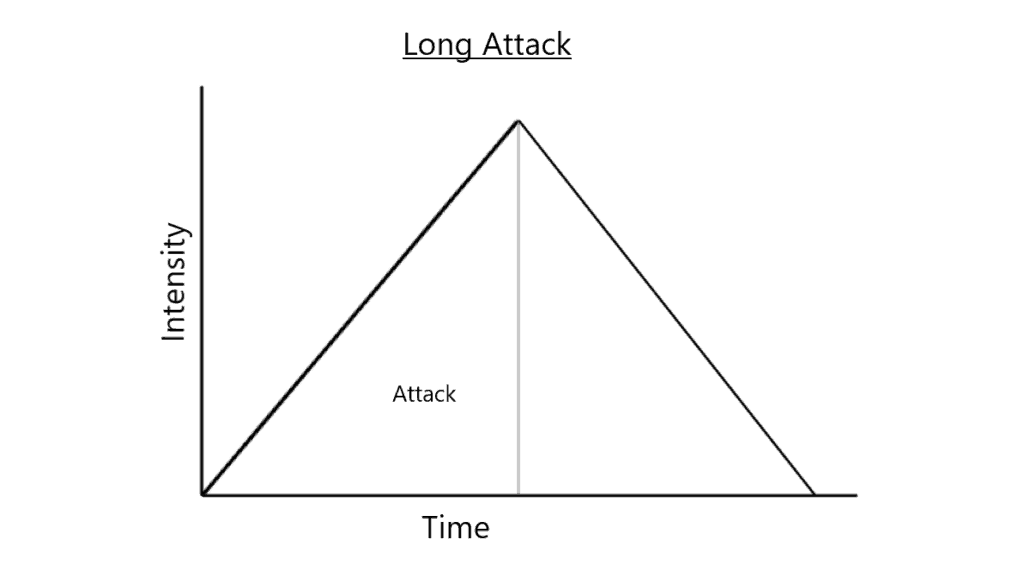
Attack is a measure of time. It represents the time it takes a note to reach its maximum intensity. 

Instruments with short attack include percussion, piano, and plucked string instruments such as guitar or harpsichord. When a note is played on these instruments, the sound quickly reaches its maximum level. 

Instruments capable of long attack include woodwinds, brass, and bowed string instruments such as violins. Notes played on these instruments can start quiet and slowly build to their maximum level.

The following graphs show a comparison between short attack and long attack:

Sustain

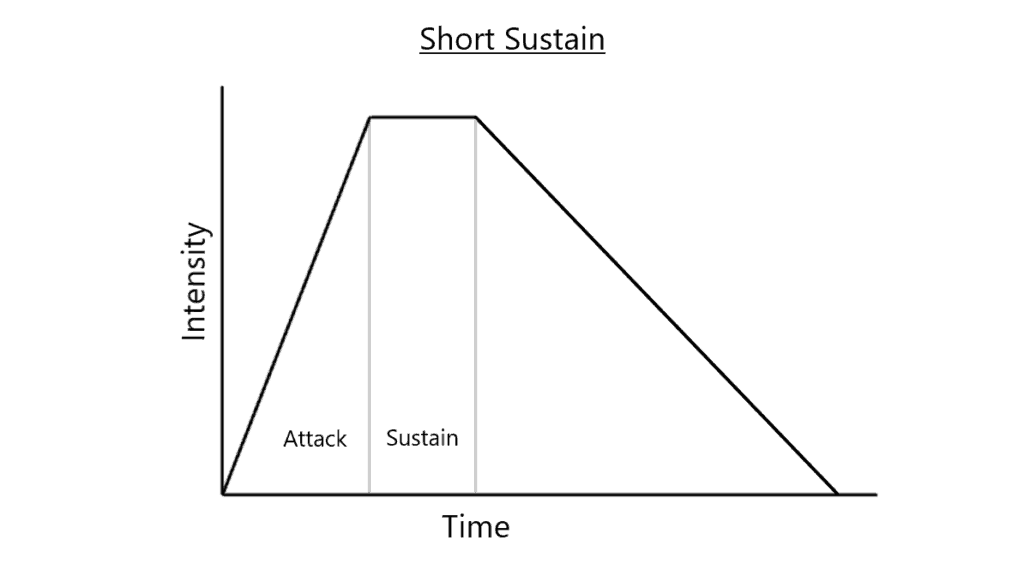
Sustain is a measure of time, in the context of acoustic instruments. It represents the duration of time that the steady-state intensity of a note is held. 

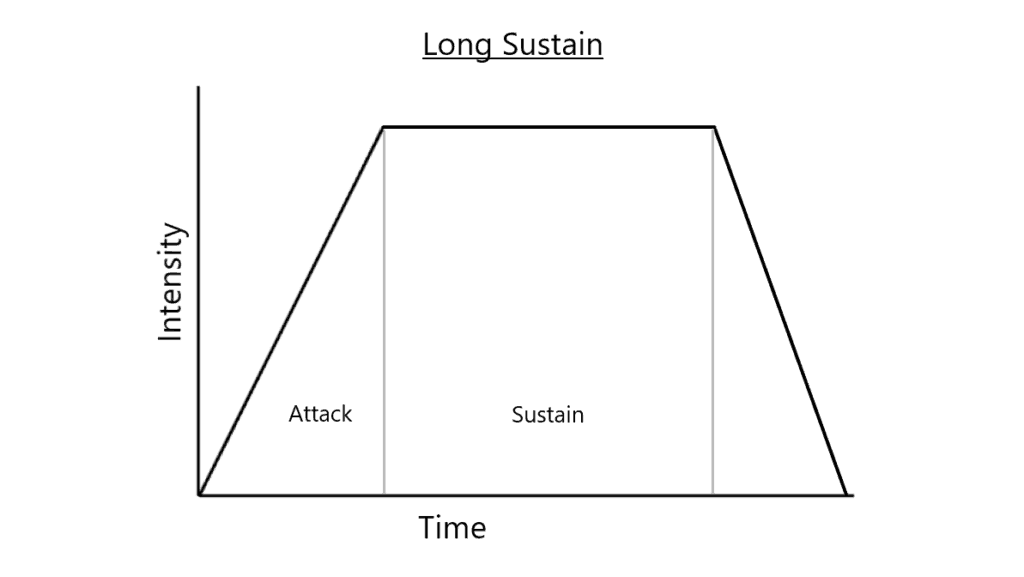
Snare drums, banjos, and many other percussive instruments are examples of instruments with short sustain. Once the note played and reaches its maximum intensity, it quickly begins to decay.

Instruments such as electric guitar, bowed string instruments, and wind instruments are capable of long sustain. The musician can hold a note for a sustained period of time at a steady-state intensity.

These graphs show a comparison between short sustain and long sustain:

Decay

Decay is a measure of time. It represents the duration of time a note takes to fall from the steady-state intensity to complete silence. 

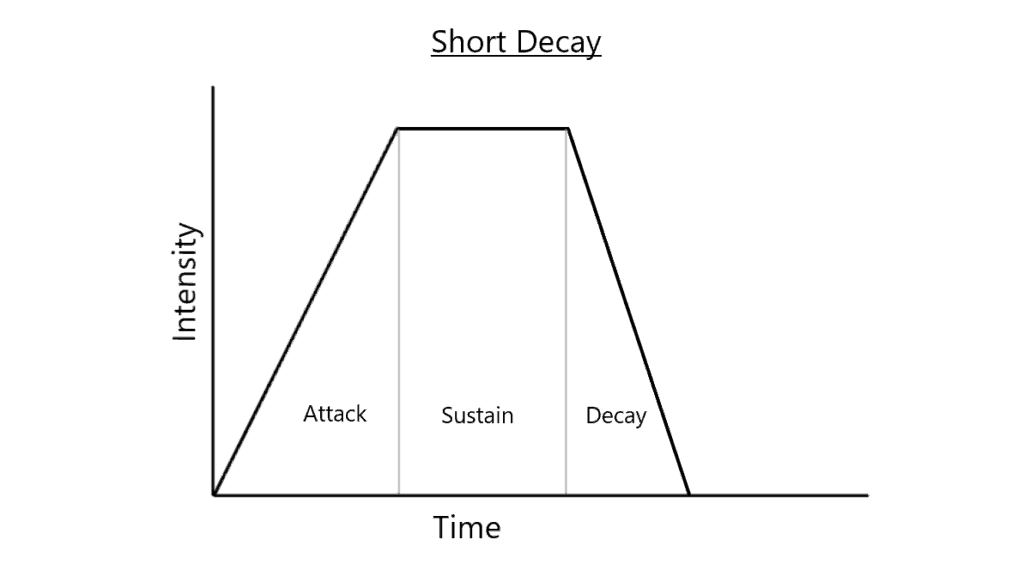
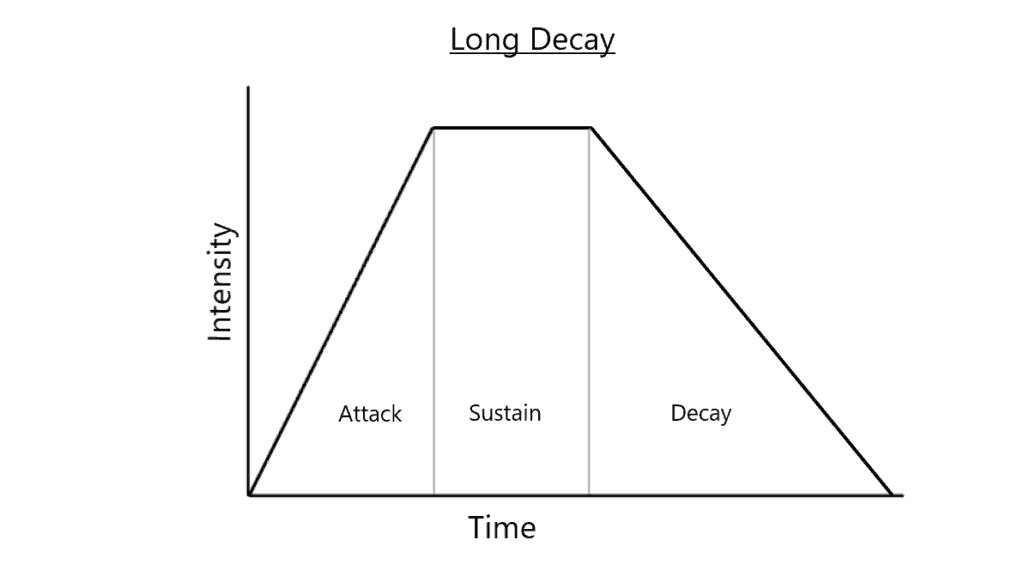
Most percussion instruments have a short decay. The intensity of the note quickly falls after it the sustain period. Even if the instrument has a long sustain time, it can have a short decay time. For example, a violinist can hold a long, sustained note and then abruptly silence the instrument.

Cymbals are one example of an instrument with a long decay time. After the initial strike, the intensity of a cymbal’s sound slowly falls until it is completely silent. Note that the musician playing the cymbal could shorten the decay significantly by silencing the cymbal with his or her hand.

The following graphs show a comparison between short decay and long decay:

The Musician Behind The Instrument

It may seem obvious, but even the same note played on the same instrument might sound different depending on who is playing it. Instruments are ultimately a tool for expression and musical technique plays a critical role in the sound produced by that tool.

It is easy to become obsessed with collecting a specific set of gear to get the perfect sound, but we must not forget the most important variable: the human behind the gear.