What is Sound?
Sound is the audible vibration of a material – the vibration of wood, metal, water, or any other material. Most often, the sounds we hear are the result of vibrating air particles. More accurately, sound is the result of fluctuating waves of positive and negative air pressure, called acoustical energy.
Sound is the result of fluctuating waves of positive and negative air pressure, called acoustical energy.
Phases of a Sound Wave
Think of a pebble dropped into a pond. The water is forced downward and then rebounds upward. This causes concentric circles to radiate outward, as seen in this drawing. Rings form as the water level rises and falls.
These .GIFs were created by Dr. Dan Russell, who’s work can be found here.

Each downward movement is followed by an upward movement. You can see this by looking at the second image, which shows the ripples across the surface of the water from the side. Over time, the waves will become less extreme and eventually the vibration will end when the energy has been depleted.

Although the above images represent waves travelling across the surface of water, something similar happens as sound waves travel through the air.
Sound waves are made up of two basic phases: Compression and Rarefaction.
Compression & Rarefaction
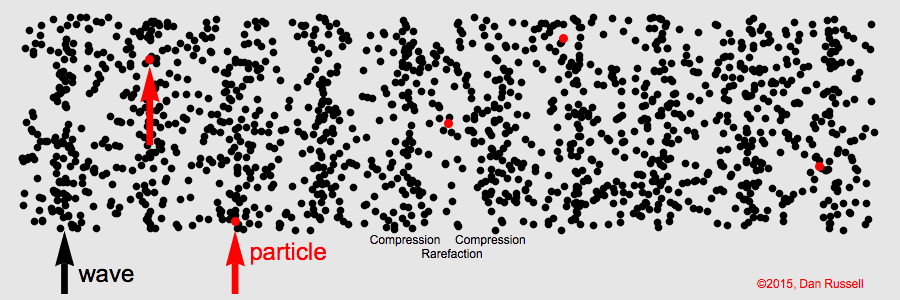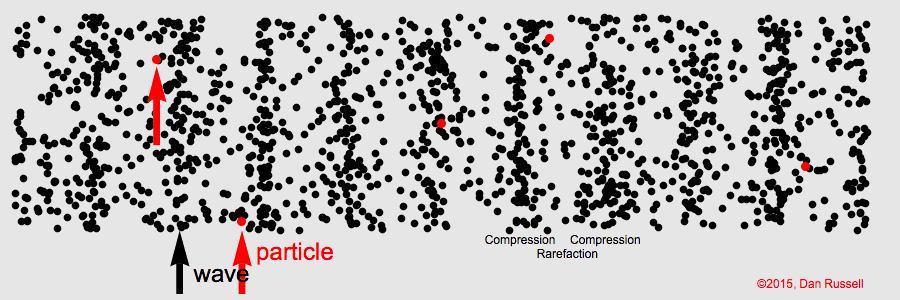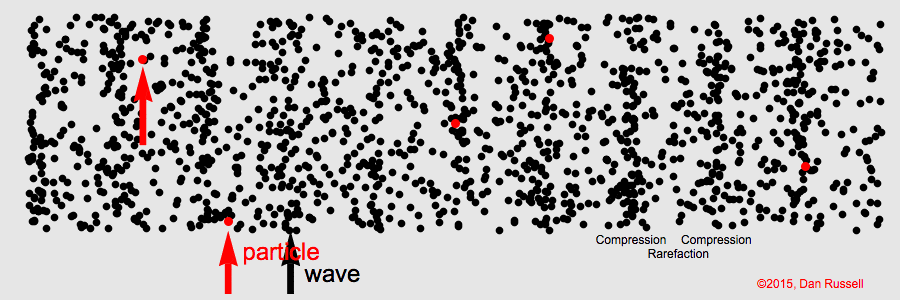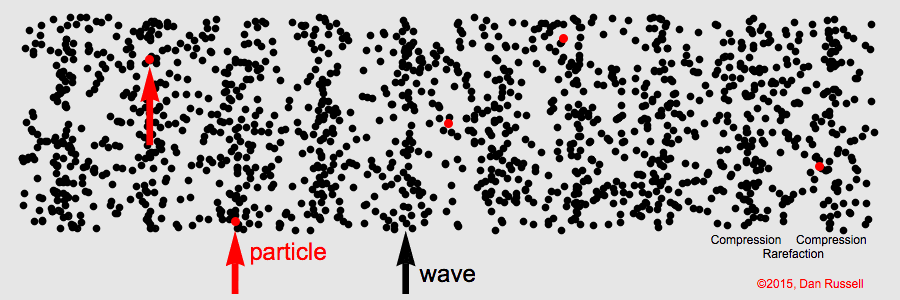
To visualize the movement of sound waves through air particles, let’s imagine a snare drum. Use this series of pictures as a reference.
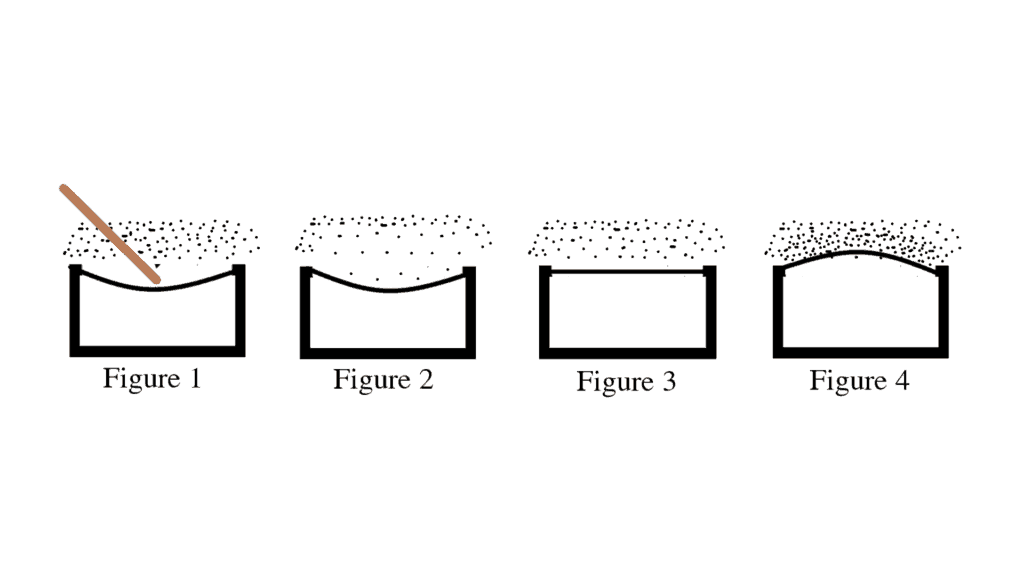
When the drummer strikes the drum from above, the drumstick forces the membrane of the drum downward, as seen in Figure 1.
The air that surrounds the membrane rushes to fill the new space, creating an area of low pressure as the air particles spread out. This is called a rarefaction, as the air molecules are rarefied (or “made rare”). This is illustrated in Figure 2.
Rarefaction occurs when the relative air pressure is decreased.
Just as in the pond example, membrane’s downward movement is followed by an upward recoiling movement, shown in Figure 3.
As the membrane moves upward, it forces the air molecules around it to bunch together, or compress. Figure 4 shows this compression.
Compression occurs when relative pressure is increased.
How Sound Waves Travel
The movement of the drum membrane not only affects the air molecules surrounding it, but it causes a chain reaction. Just like the example of the pebble in the pond, sound waves ripple outward until the air pressure settles back to equilibrium.
Look at these .GIFs created by Dr. Daniel A. Russell, found at this link. They are excellent tools for visualizing the chain reaction that occurs as a sound wave travels through the air.

When you hear the sound of a snare drum or any sound source, you are really hearing the vibrations in the air that the snare drum causes. The drum doesn’t vibrate your eardrums directly, nor do the particles of air that surround the drum.
Using Dr. Russell’s next GIF, you can see that the individual particles of air simply move back and forth. The sound wave that reaches your ear is the result of energy being transferred from one particle to another. The particles are barely displaced.
Inverse Square Law
In the ‘pebble causing ripples across the surface of the water’ example, we saw the waves as they expanded outward from the source in two dimensions, forming rings.
If unobstructed, sound radiates outward from the source in three dimensions, creating a sphere of acoustical energy.
As the wave travels away from the source, its intensity is attenuated, or reduced. The reduction sound intensity over distance is described by the inverse square law. With every doubling of distance, intensity is decreased by four times.
The inverse square law predicts that sound intensity level will be decreased as the surface area of the sphere of acoustical energy expands.
For more information, read this article on the inverse square law by Audio University.
Speed of Sound
The speed of sound is determined by the material carrying the wave.
For example, sound travels more slowly in water than it does in air. The temperature of the air also has an effect on the speed of sound.
For simplicity’s sake, the standard speed of sound is 1130 feet/second (344 meters/second). This is based on the speed of a sound wave on a standard temperature day at 59° Fahrenheit (15° Celsius).
Sound waves at 1130 ft/s travel 1 foot every 1.1 millisecond.
Through a given material, all sounds will travel at the same speed regardless of their properties.
Properties of a Sound Wave
Now that you understand what sound waves are, let’s take a closer look at the properties that we use to describe them. In this section, you’ll learn about phase, frequency, amplitude, period, and wavelength.
Phase
Sound waves are cycles of positive and negative pressure changes. Phases of increasing pressure are followed by phases of decreasing pressure, and so on.
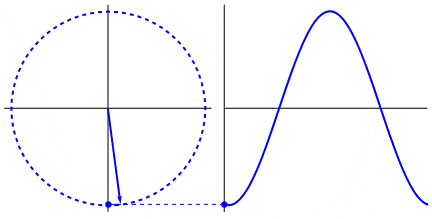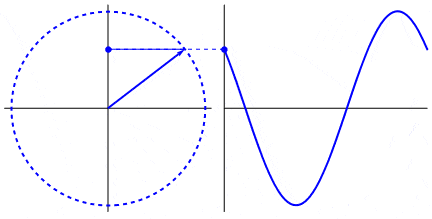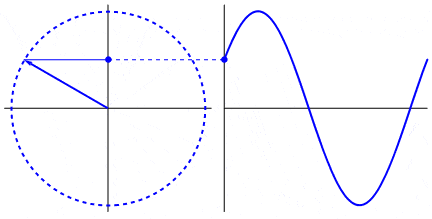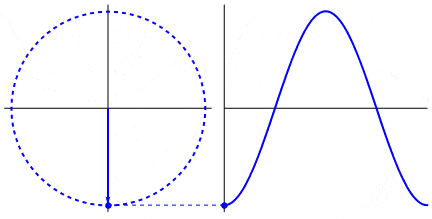
If you draw a sound wave on a graph, the graph will show a circle. The moments of maximum compression are represented at the circle’s highest point and the moments of maximum rarefaction are represented at the lowest point of the circle.
The phase of a wave describes the various points along the perimeter of the circle, or along the progression of the wave.
If time is added to the graph, the circle becomes a sine wave. This .GIF that was posted by ‘Andy aka’ in a StackExchange forum is the perfect tool for visualizing this. On this graph, the y-axis still shows the moments of maximum compression at the top and maximum rarefaction at the bottom. However, now the x-axis shows the progression of time from right to left.
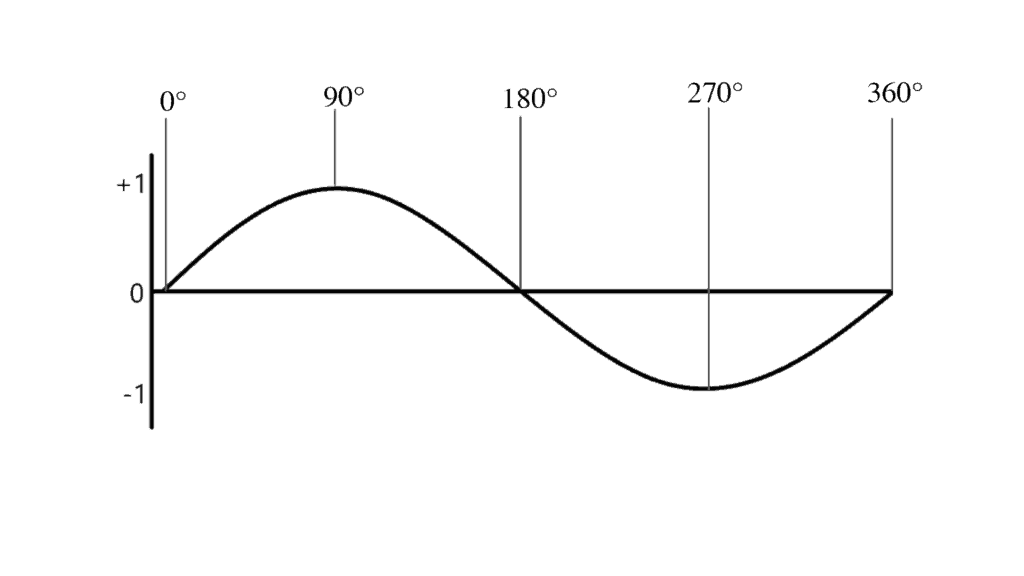
Degrees (0° – 360°)
Phase is measured in degrees.
A sound wave is a circular motion, so its phase is described within the range of 0°-360°.
The zero-degree starting point can be anywhere along the wave.
In this graph, the zero-degree point is at the start of the compression phase as the line intersects with the x-axis (heading upward). The 90-degree point is at the height of the compression phase. The line heads downward, crossing the x-axis again (180-degree point). The 270-degree point is at the lowest point on the graph where the rarefaction is most extreme.
Frequency
One 360-degree revolution is called a cycle. Sound waves oscillate very quickly, completing many cycles of high and low pressure per second.
The frequency of a sound wave describes how many cycles are completed per second.
Humans are capable of hearing sounds between 20 cycles per second and 20,000 cycles per second!
Frequency and Pitch
Frequency corresponds to musical pitch, although pitch is also affected by other factors.
Generally speaking, the higher the frequency, the higher the perceived pitch.
For example, doubling the frequency of a sound increases its pitch by one octave. If the frequency is reduced by half, the pitch of the sound will be one octave lower.
Hertz (Hz)
Frequency is measured in Hertz, abbreviated Hz. Hertz is a unit of measurement that represents cycles per second.
One cycle per second is 1 Hz, or one Hertz. One thousand cycles per second is 1 kHz, or one kilohertz.
As stated above, humans are capable of hearing sounds between 20 to 20,000 cycles per second. Written in Hertz, the human range of hearing is 20 Hz and 20 kHz.
Period
As you learned above, frequency is the number of complete cycles within one second.
Period is the amount of time it takes a wave to complete one 360-degree cycle.
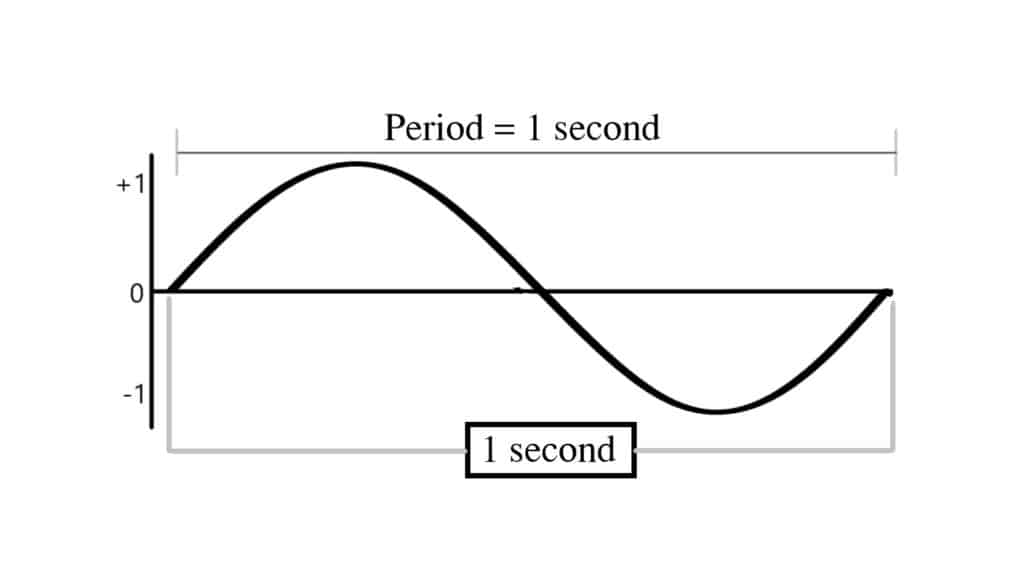

Period and Time
The period of a wave is a measurement of time, usually represented in seconds or milliseconds.
The period of a 1 Hz sound wave is 1 second. The period of a 2 Hz sound wave is 0.5 seconds.
As you get into the audible frequency range, milliseconds become more useful than seconds. A 20 Hz sound wave has a period of 50 milliseconds.
The formula used to calculate the period in seconds is very simple: Period (s) = 1 ÷ Frequency.
If you are trying to find the period in milliseconds, use this formula: Period (ms) = 1000 ÷ Frequency.
Try to find the period of the following frequencies and check your work with this chart:
| Frequency | Period (in seconds) | Period (in milliseconds) |
|---|---|---|
| 100 Hz | 0.01 s | 10 ms |
| 1 kHz | 0.001 s | 1 ms |
| 10 kHz | 0.0001 s | 0.1 ms |
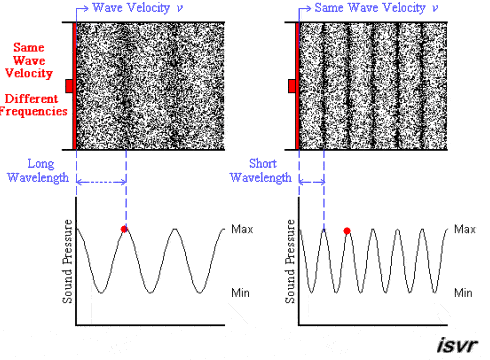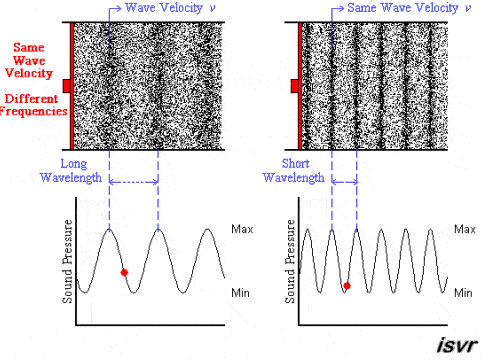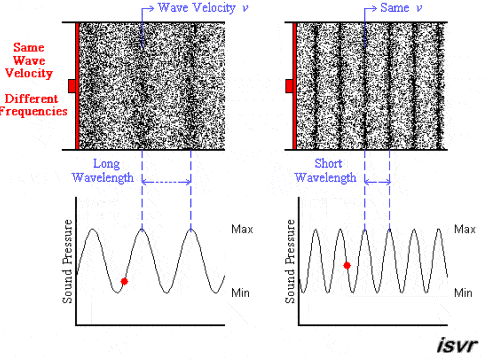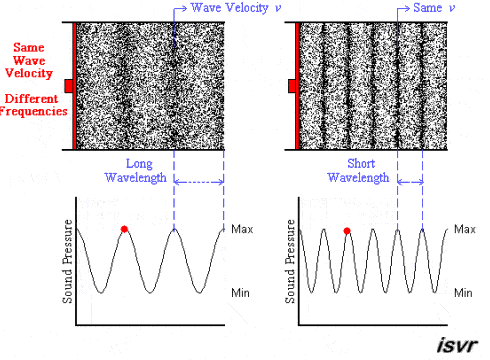
Wavelength
Wavelength is often confused with period, but they are very different. Period is a measurement of time, whereas wavelength is a measurement of distance.
The wavelength of a frequency describes the physical distance it takes to complete one cycle.

Higher frequencies have shorter wavelengths, whereas lower frequencies have longer wavelengths.
You can see this animated in the following .GIF, created by the Institute of Sound and Vibration Research (ISVR). This work is extremely helpful for understanding the relationship between frequency and wavelength.
Wavelength and Distance
As mentioned above, wavelength changes with the speed of sound. The speed of sound changes with the density of the particles carrying the sound wave.
To calculate the wavelength of a given frequency, use this formula:
Wavelength = Speed of Sound / Frequency
Although differences in temperature and humidity can have an effect on this calculation, using the standard 1130 ft./second speed of sound will give you very close to an accurate result.
Amplitude
Amplitude describes the extent of the pressure changes. Higher amplitude waves are waves with more extreme compression and rarefaction. Lower amplitude waves are waves with less extreme pressure changes.
Amplitude describes the extent of the force caused by a sound wave.
Amplitude and Loudness
Amplitude correlates to the loudness of a sound wave. It’s the part of a sound wave that is measured on the vertical y-axis on this graph.
The higher the amplitude, the louder the sound. The lower the amplitude, the quieter the sound.
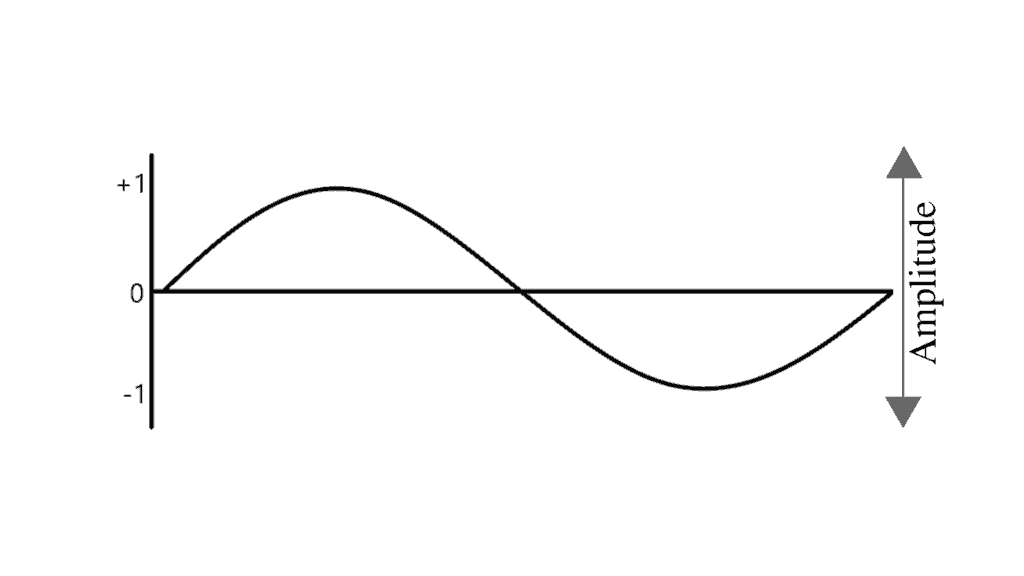
The centerline of the graph represents zero amplitude, meaning normal air pressure. As the waveform travels further above or below the centerline, the amplitude grows. There can be positive amplitude and negative amplitude.
During the transition between each compression and rarefaction, the waveform crosses the centerline. This means that there is a brief moment of zero amplitude, or normal pressure level, in each transition between positive and negative.
Decibels (dB SPL)
The unit used to measure air pressure is the pascal. However, we usually describe sound pressure levels using decibels instead.
Decibels are the unit used to measure the ratio of change in the amplitude of a sound wave. The abbreviation for decibels of sound pressure level is dB SPL.
The reason decibels are used instead of pascals is because humans are capable of hearing such a vast range of amplitude. Pascals are a linear unit of measurement, whereas decibels are a logarithmic unit of measurement. The best way to understand what this means is to compare the range of human hearing written in pascals and dB SPL.
The following chart shows the range of human hearing using pascals in the first column, while the second column uses decibels. The values are written from least extreme to most extreme.
| Sound Source Level (1 meter) | Sound Pressure Level (in Pascals) | Sound Pressure Level (in Decibels) |
|---|---|---|
| Threshold of Hearing (Quietest Sound) | 0.00002 Pa | 0 dB SPL |
| Speech | 0.02 Pa | 60 dB SPL |
| Music | 0.2 Pa | 80 dB SPL |
| Threshold of Pain (Extremely Loud) | 20 Pa | 120 dB SPL |
The human range of hearing ranges from 0.00002 Pa to 200 Pa! These numbers are not very convenient for calculation and communication. Using the logarithmic scale of decibels, however, provides much more manageable numbers for our minds to understand.
It’s a bit confusing in the beginning. So if you remember one thing about dB SPL, remember this: adding 6dB doubles the sound pressure level and subtracting 6dB cuts the sound pressure level in half.
After a while, you’ll get a hang of it. If you’re new to this way of thinking (like I was in the beginning), it’s normal to feel like you don’t completely understand. Don’t sweat it! I’ll be sure to go over decibels in a future post for those who are interested in learning more.
Start with the Basics
If there is one thing I can recommend learning to become successful in the audio production field, it is to learn the basics. If you learn why things work, you’ll be able to figure out how things work. The truth is, you will be dealing with new equipment everyday in constantly changing situations. Learning the concepts that are the foundation of everything in audio will allow you to respond intelligently to any scenario you might face.
As always, I want you to know that I really appreciate your interest in these articles and the time you are putting into growing your own understanding of audio production.
